Understanding Perpendicular Lines and Their Slopes
Understanding Perpendicular Lines and Their Slopes

Perpendicular lines are a fundamental concept in geometry, often appearing in various mathematical and real-world applications. Whether you’re a student tackling geometry problems or a professional working with design and engineering, grasping the concept of perpendicular lines and their slopes is crucial. This guide will walk you through everything you need to know, from the basics to practical applications.
What Are Perpendicular Lines?
Perpendicular lines are lines that intersect at a 90-degree angle (right angle). This relationship is a cornerstone in geometry, influencing everything from construction to computer graphics. Understanding perpendicularity helps in identifying and creating precise angles in various scenarios.
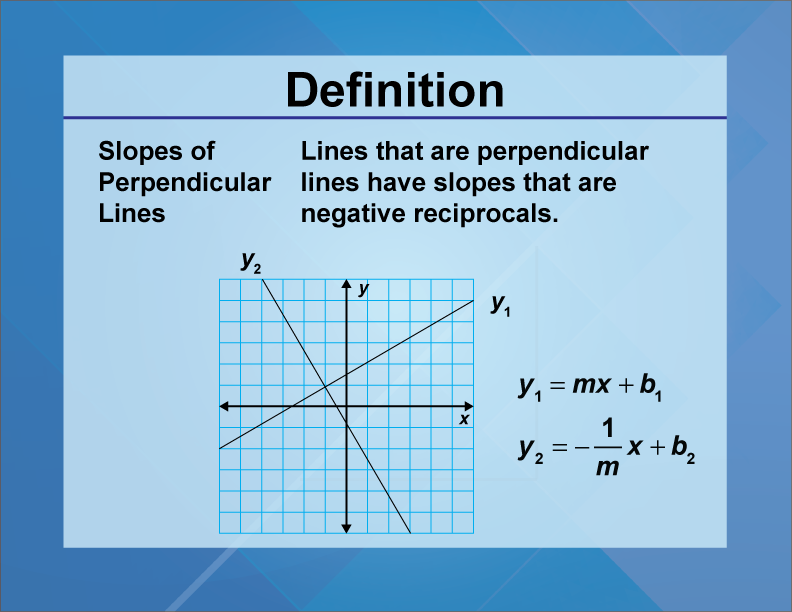
The Role of Slopes in Perpendicular Lines
The slope of a line measures its steepness and direction. For two lines to be perpendicular, their slopes must satisfy a specific condition: the product of their slopes must be -1. Mathematically, if the slope of one line is ( m_1 ) and the slope of the other line is ( m_2 ), then:
[ m_1 \times m_2 = -1 ]
This relationship is essential for determining perpendicularity algebraically.
How to Find the Slope of a Perpendicular Line
To find the slope of a line perpendicular to a given line, follow these steps:
- Identify the slope of the given line: Let’s say the slope is ( m ).
- Calculate the negative reciprocal: The slope of the perpendicular line is ( -\frac{1}{m} ).
📘 Note: If the given line is vertical (undefined slope), the perpendicular line will be horizontal (slope = 0), and vice versa.
Practical Applications of Perpendicular Lines
Perpendicular lines are not just theoretical; they have real-world applications in:
- Architecture: Ensuring walls are plumb and floors are level.
- Engineering: Designing structures with precise angles for stability.
- Graphics Design: Creating grids and layouts with right angles.
Checklist for Working with Perpendicular Lines
- Identify the slopes of the lines in question.
- Check if the product of the slopes equals -1.
- Use the negative reciprocal to find the slope of a perpendicular line.
- Verify the angle between the lines is 90 degrees.
Wrapping Up

Understanding perpendicular lines and their slopes is a vital skill in geometry and its applications. By mastering the relationship between slopes and the concept of negative reciprocals, you can confidently tackle problems involving perpendicularity. Whether you’re studying for an exam or working on a project, this knowledge will prove invaluable.
What is the definition of perpendicular lines?
+Perpendicular lines are lines that intersect at a 90-degree angle (right angle).
How do you determine if two lines are perpendicular?
+Two lines are perpendicular if the product of their slopes is -1.
What is the slope of a line perpendicular to a horizontal line?
+A horizontal line has a slope of 0, so a line perpendicular to it is vertical and has an undefined slope.
Can two vertical lines be perpendicular?
+No, two vertical lines are parallel, not perpendicular, as they never intersect.
Why are perpendicular lines important in real-world applications?
+Perpendicular lines ensure precision in construction, engineering, and design, contributing to stability and accuracy.
perpendicular lines, slopes, geometry, negative reciprocal, right angle,



